Writing Numbers In Scientific Notation (Examples)

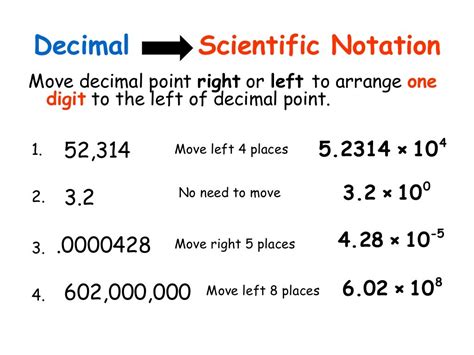
Numbers can sometimes be very large or very small, making them difficult to work with or comprehend. To simplify these numbers, scientists and mathematicians use scientific notation. Scientific notation allows us to express numbers in a concise and standardized way. In this article, we will explore how to write numbers in scientific notation with the help of examples and explanations.

What is Scientific Notation?

Scientific notation is a way of expressing numbers as a product of a coefficient and a power of 10. It is commonly used in scientific and mathematical fields to represent very large or very small numbers. The general form of a number in scientific notation is:

a x 10^b

Where a is a decimal number between 1 and 10 (inclusive) and b is an integer that represents the power of 10. The number a is sometimes referred to as the coefficient or mantissa, and the number b is the exponent.

Writing Numbers in Scientific Notation

Let’s take a look at some examples to understand how to write numbers in scientific notation:

Example 1: Large Numbers

Consider the number 3,000,000. To write this number in scientific notation, we need to move the decimal point to the left until we have a number between 1 and 10. In this case, we can move the decimal point 6 places to the left:

3,000,000 = 3.0 x 10⁶

Similarly, the number 25,000,000 can be written as:

25,000,000 = 2.5 x 10⁷

Example 2: Small Numbers

Now let’s consider a small number, such as 0.000045. To write this number in scientific notation, we need to move the decimal point to the right until we have a number between 1 and 10. In this case, we can move the decimal point 5 places to the right:

0.000045 = 4.5 x 10^-5

Similarly, the number 0.00000028 can be written as:

0.00000028 = 2.8 x 10^-7

Example 3: Calculating Exponents

When converting a number to scientific notation, it is important to determine the correct exponent. To do this, count the number of places the decimal point was moved. If the decimal is moved to the left, the exponent is positive. If the decimal is moved to the right, the exponent is negative.

For example, let’s convert 540,000 to scientific notation:

We can move the decimal point 5 places to the left:

540,000 = 5.4 x 10⁵

Let’s convert 0.0000038 to scientific notation:

We can move the decimal point 6 places to the right:

0.0000038 = 3.8 x 10^-6

Conclusion

Writing numbers in scientific notation allows us to express very large or very small numbers in a standardized and concise way. By moving the decimal point and determining the correct exponent, we can easily convert numbers to scientific notation. This notation is widely used in scientific and mathematical fields, enabling easier calculations and comparisons.

Frequently Asked Questions

1. Why is scientific notation used?

Scientific notation is used to represent very large or very small numbers in a concise and standardized way, making them easier to work with and comprehend.

2. How do you convert a number to scientific notation?

To convert a number to scientific notation, move the decimal point until you have a number between 1 and 10. Count the number of places the decimal point was moved to determine the exponent.

3. What does the exponent represent in scientific notation?

The exponent in scientific notation represents the power of 10 by which the coefficient should be multiplied.

4. Can scientific notation be used for any number?

Scientific notation is typically used for numbers that are very large or very small. It is not commonly used for numbers that are between 1 and 10.

5. Is scientific notation the same as exponential notation?

Scientific notation and exponential notation are similar concepts, but they are not exactly the same. Exponential notation is a more general form that can be used for any base, while scientific notation specifically uses base 10.