Coefficient Of Variation: Calculation Guide With Examples
Welcome to our comprehensive guide on calculating the coefficient of variation. Whether you’re a student, researcher, or working professional, understanding this statistical measure is essential for analyzing and comparing data. In this guide, we’ll explain what the coefficient of variation is, why it’s important, and how to calculate it. We’ll also provide examples to help solidify your understanding. Let’s dive in!
Table of Contents
- Introduction
- What is Coefficient of Variation?
- Why is Coefficient of Variation Important?
- How to Calculate Coefficient of Variation
- Example 1: Calculating Coefficient of Variation for a Single Dataset
- Example 2: Comparing Coefficient of Variation for Multiple Datasets
- Limitations of Coefficient of Variation
- Interpretation of Coefficient of Variation
- When to Use Coefficient of Variation
- Conclusion
- FAQs
Introduction
When working with data, it’s crucial to have statistical measures that allow us to compare and analyze datasets effectively. One such measure is the coefficient of variation, which provides insights into the relative variability of a dataset. In this guide, we’ll explore the concept of coefficient of variation, its calculation methods, and its applications through various examples.
What is Coefficient of Variation?
The coefficient of variation (CV) is a statistical measure that expresses the relative variability of a dataset in relation to its mean. It allows us to compare the variability of two or more datasets, even if they have different units or scales of measurement. The CV is calculated as the ratio of the standard deviation to the mean, multiplied by 100 to express it as a percentage.
Calculation Formula
The formula for calculating the coefficient of variation is:
CV = (Standard Deviation / Mean) * 100Why is Coefficient of Variation Important?
The coefficient of variation is an important statistical measure for several reasons:
- Comparing Variability: The CV allows us to compare the variability of different datasets, regardless of their units or scales of measurement. This is particularly useful when analyzing datasets with different means.
- Identifying Relative Stability: The CV provides insights into the stability or consistency of a dataset. A lower CV indicates more stability, while a higher CV suggests greater variability.
- Evaluating Risk: In finance and investment analysis, the CV is used to assess the risk associated with different investments. A lower CV indicates lower risk, while a higher CV implies higher risk.
How to Calculate Coefficient of Variation
Calculating the coefficient of variation involves the following steps:
- Calculate the mean of the dataset.
- Calculate the standard deviation of the dataset.
- Apply the formula:
CV = (Standard Deviation / Mean) * 100.
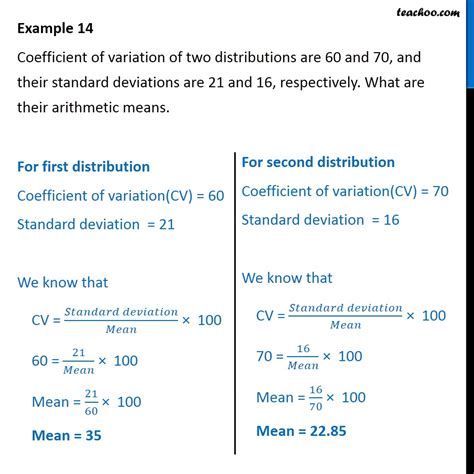
Example 1: Calculating Coefficient of Variation for a Single Dataset
Let’s say we have a dataset consisting of monthly sales figures for a company over the past year. We want to calculate the coefficient of variation to understand the relative variability of the sales data.
Step 1: Calculate the mean of the dataset by summing all the values and dividing by the number of observations.
Step 2: Calculate the standard deviation of the dataset using the appropriate statistical formula.
Step 3: Apply the formula for coefficient of variation: CV = (Standard Deviation / Mean) * 100.
For example, if the mean is 500 and the standard deviation is 50, the coefficient of variation would be:
CV = (50 / 500) * 100 = 10%
Example 2: Comparing Coefficient of Variation for Multiple Datasets
Now, let’s consider a scenario where we want to compare the variability of two different datasets: the monthly sales figures for two different companies.
Step 1: Calculate the mean of each dataset separately.
Step 2: Calculate the standard deviation of each dataset.
Step 3: Apply the formula for coefficient of variation to each dataset.
For example, if Company A has a mean of 500 and a standard deviation of 50, while Company B has a mean of 1000 and a standard deviation of 100, the coefficients of variation would be:
CV for Company A = (50 / 500) * 100 = 10%CV for Company B = (100 / 1000) * 100 = 10%
Based on the coefficients of variation, both datasets exhibit the same relative variability, despite having different means and standard deviations.
Limitations of Coefficient of Variation
While the coefficient of variation is a useful statistical measure, it does have some limitations:
- Dependence on Mean: The CV is influenced by the mean of the dataset. If the mean is close to zero or very small, the CV can become artificially large.
- Unit Dependency: The CV is sensitive to the units or scales of measurement used in the dataset. This makes it challenging to compare datasets with different units.
- Applicability to Non-Numeric Data: The CV is primarily designed for numeric data and may not be applicable to non-numeric data types.
Interpretation of Coefficient of Variation
The interpretation of the coefficient of variation depends on the context and the specific dataset being analyzed. However, the following general guidelines can be used:
- A CV of 0% indicates no variability, meaning all values are the same.
- A CV of less than 10% is considered low variability.
- A CV between 10% and 30% is considered moderate variability.
- A CV greater than 30% is considered high variability.
When to Use Coefficient of Variation
The coefficient of variation is useful in various fields and applications, including:
- Finance and Investment: The CV helps assess the risk associated with different investments, allowing investors to make informed decisions.
- Economics: The CV is used to analyze economic data and compare the variability of different economic indicators.
- Quality Control: The CV is used in quality control processes to evaluate the consistency and stability of manufacturing processes.
- Research and Data Analysis: The CV is a valuable tool for researchers and data analysts to understand the relative variability of datasets.
Conclusion
The coefficient of variation is a powerful statistical measure that allows us to compare the relative variability of different datasets. By calculating the CV, we gain insights into the stability, risk, and consistency of the data. Understanding how to calculate and interpret the coefficient of variation is essential for making informed decisions in various fields. Hopefully, this guide has provided you with a comprehensive understanding of the coefficient of variation and its applications.
